Combinatorics, Graph Theory and Applications. COMBGRAF

Advanced Course: Combinatorial Convexity (May 7-18, 2012)



Combinatorics, Graph Theory and Applications


Doctoral Program on Applied Mathematics


Universitat Politècnica de Catalunya




Advanced Course


Combinatorial Convexity


by Imre Bárány



May 7-18, 2012






Course Description


The course will cover several topics in combinatorial convexity, where  theorems of Caratheodory, Helly, Radon, and Tverberg are the typical and  classical results. We plan to investigate weak epsilons-nets, halving  lines and planes, the (p,q) problem and its solution, extensions to lattice convex sets, and colourful versions of theorems of Helly,  Caratheodory, Radon, Tverberg, and the like. Further possible  topics are transversals, lattice polytopes and random polytopes. The  methods here use tools from linear algebra, combinatorics, topology,  geometry, probability theory, and geometry of numbers.

Some literature:

 

  • the classic survey by Danzer, Grunbaum, Klee "Helly's  theorem and its relatives", Convexity, Proc. Symp. Pure Math., 7, American Mathematical Society, pp. 101–179.
  • some chapters from J. Matousek's excellent  book "Lectures on discrete geometry" Springer GTM Vol. 212, 2002.


Imre Bárány


Imre Bárány is a member of the Alfred Rényi Institute of Mathematics in Budapest and of the University College of London. His main research interests are in combinatorics and discrete geometry. Among his main contributions, he gave a surprisingly simple alternative proof of Lovász theorem on the chromatic number of Kneser graphs, he solved a problem of Sylvester on the probability of random point sets in convex position, he gave colored versions of Caratheodoy and Helly  theorems and proved a central limit theorem on random points in convex bodies. He received the Erdös prize of the Hungarian Academy of Sciences in 1982, was invited speaker in the ICM 2002 held in Beijing and he is in the Editorial Board of several journals, including Combinatorica, Mathematika, and the Online Journal of Analytic Combinatorics.


Registration and further information


Please send an e-mail tagged 'Barany2012Course' to convexitycourse2012@ma4.upc.edu indicating

Name
Affiliation
e-mail address
Short cv (academic degree, current situation, mathematical background)

 

There is no registration fee.

For further information please contact convexitycourse2012@ma4.upc.edu.

Poster announcement of the course.


Schedule


Monday May 7 10h Welcome coffee (room 'R', FME)
11h-13h
Tuesday May 8 11h-13h Tuesday May 15 11h-13h
Wednesday May 9 11h-13h Wednesday May 16 11h-13h
Thursday May 10 11h-13h Thursday May 17 11h-13h
Friday May 11 11h-13h


Preliminary list of participants


Aguiló, Francesc (Ma4, UPC Barcelona)

Balbuena, Camino  (UPC, Barcelona)

Ball, Simeon (Ma4, UPC, Barcelona)

Boroczky, Karoly (Renyi Institute Budapest)

Brunat, Josep M. (Ma2, UPC, Barcelona)

Comellas, Francesc (Ma4, UPC, Barcelona)

Dalfó, Cristina (Ma4, UPC, Barcelona)

Fàbrega, Josep (Ma4, UPC, Barcelona)

Fiol, Miquel Àngel (Ma4, UPC, Barcelona)

Hansberg, Adriana (UPC, Barcelona)

Huemer, Clemens (Ma4, UPC, Barcelona)

Lladó, Anna (Ma4, UPC, Barcelona)
López-Masip, Susana-Clara (Ma4, UPC, Barcelona)

Magazinov, Alexander (Mathematical Institute of RAS, Moscow)

Maureso, Montserrat (Ma2, UPC, Barcelona)

Miralles, Alícia (Ma4, UPC, Barcelona)

Molina, Enrique (UPC, Barcelona)

Mora, Mercè (Ma2, UPC Barcelona)

Padrol, Arnau (Ma2, UPC, Barcelona)
Pelayo, Ignacio (Ma3, UPC Barcelona)
Perarnau, Guillem (Ma4, UPC, Barcelona)
Pérez-Mansilla, Sónia (Ma4, UPC, Barcelona)
Pfeifle, Julian (Ma2, UPC, Barcelona)
Petridis, Giorgis (Cambridge University)
Serra, Oriol (Ma4, UPC, Barcelona)


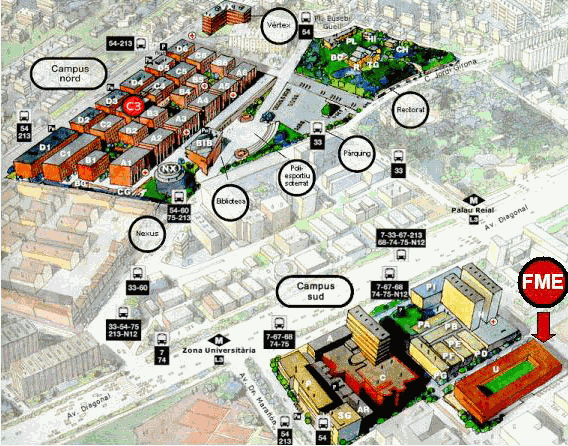
Lecture room and directions


The lectures will be held in Room 100 in the building of the Facultat de Matemàtiques i Estadística (FME).

 

Please see the map for the location of the building. To get there you can use Metro L3 (Green Line)

and step off at Palau Reial. For further directions ask info.osrm@upc.edu.

 



Updates will be posted concerning lecture room, directions, list of participants and course notes.